README_EN.md

comments	difficulty	edit_url	rating	source	tags
true	Medium	https://github.com/doocs/leetcode/edit/main/solution/1000-1099/1035.Uncrossed%20Lines/README_EN.md	1805	Weekly Contest 134 Q3	Array Dynamic Programming

1035. Uncrossed Lines

中文文档

Description

You are given two integer arrays nums1 and nums2. We write the integers of nums1 and nums2 (in the order they are given) on two separate horizontal lines.

We may draw connecting lines: a straight line connecting two numbers nums1[i] and nums2[j] such that:

• nums1[i] == nums2[j], and
• the line we draw does not intersect any other connecting (non-horizontal) line.

Note that a connecting line cannot intersect even at the endpoints (i.e., each number can only belong to one connecting line).

Return the maximum number of connecting lines we can draw in this way.

 

Example 1:

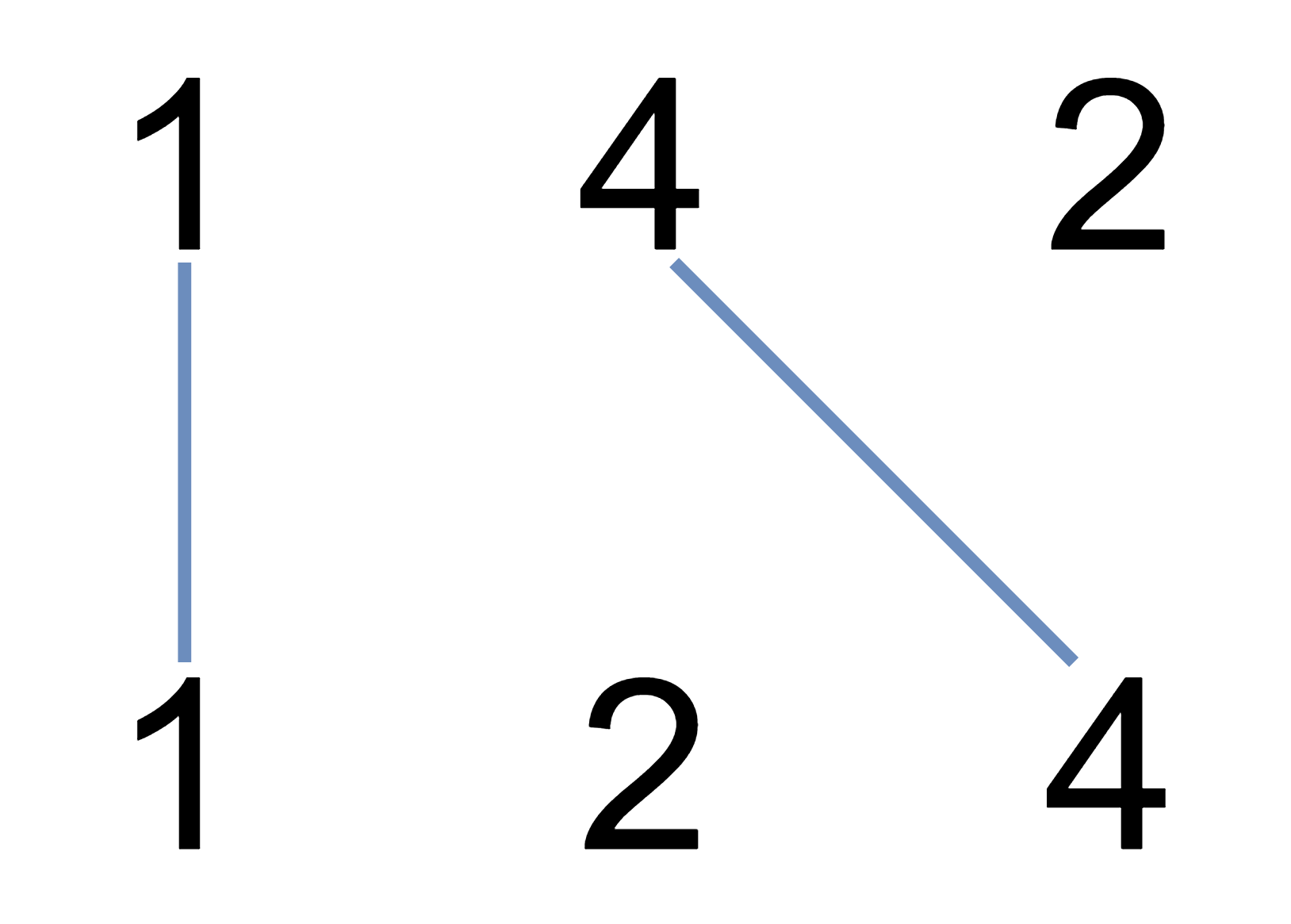
Input: nums1 = [1,4,2], nums2 = [1,2,4]
Output: 2
Explanation: We can draw 2 uncrossed lines as in the diagram.
We cannot draw 3 uncrossed lines, because the line from nums1[1] = 4 to nums2[2] = 4 will intersect the line from nums1[2]=2 to nums2[1]=2.

Example 2:

Input: nums1 = [2,5,1,2,5], nums2 = [10,5,2,1,5,2]
Output: 3

Example 3:

Input: nums1 = [1,3,7,1,7,5], nums2 = [1,9,2,5,1]
Output: 2

 

Constraints:

• 1 <= nums1.length, nums2.length <= 500
• 1 <= nums1[i], nums2[j] <= 2000

Solutions

Solution 1: Dynamic Programming

We define $f[i][j]$ to represent the maximum number of connections between the first $i$ numbers of $\textit{nums1}$ and the first $j$ numbers of $\textit{nums2}$. Initially, $f[i][j] = 0$, and the answer is $f[m][n]$.

When $\textit{nums1}[i-1] = \textit{nums2}[j-1]$, we can add a connection based on the first $i-1$ numbers of $\textit{nums1}$ and the first $j-1$ numbers of $\textit{nums2}$. In this case, $f[i][j] = f[i-1][j-1] + 1$.

When $\textit{nums1}[i-1] \neq \textit{nums2}[j-1]$, we either solve based on the first $i-1$ numbers of $\textit{nums1}$ and the first $j$ numbers of $\textit{nums2}$, or solve based on the first $i$ numbers of $\textit{nums1}$ and the first $j-1$ numbers of $\textit{nums2}$, taking the maximum of the two. That is, $f[i][j] = \max(f[i-1][j], f[i][j-1])$.

Finally, return $f[m][n]$.

The time complexity is $O(m \times n)$, and the space complexity is $O(m \times n)$. Here, $m$ and $n$ are the lengths of $\textit{nums1}$ and $\textit{nums2}$, respectively.

Python3

class Solution:
    def maxUncrossedLines(self, nums1: List[int], nums2: List[int]) -> int:
        m, n = len(nums1), len(nums2)
        f = [[0] * (n + 1) for _ in range(m + 1)]
        for i, x in enumerate(nums1, 1):
            for j, y in enumerate(nums2, 1):
                if x == y:
                    f[i][j] = f[i - 1][j - 1] + 1
                else:
                    f[i][j] = max(f[i - 1][j], f[i][j - 1])
        return f[m][n]

Java

class Solution {
    public int maxUncrossedLines(int[] nums1, int[] nums2) {
        int m = nums1.length, n = nums2.length;
        int[][] f = new int[m + 1][n + 1];
        for (int i = 1; i <= m; ++i) {
            for (int j = 1; j <= n; ++j) {
                if (nums1[i - 1] == nums2[j - 1]) {
                    f[i][j] = f[i - 1][j - 1] + 1;
                } else {
                    f[i][j] = Math.max(f[i - 1][j], f[i][j - 1]);
                }
            }
        }
        return f[m][n];
    }
}

C++

class Solution {
public:
    int maxUncrossedLines(vector<int>& nums1, vector<int>& nums2) {
        int m = nums1.size(), n = nums2.size();
        int f[m + 1][n + 1];
        memset(f, 0, sizeof(f));
        for (int i = 1; i <= m; ++i) {
            for (int j = 1; j <= n; ++j) {
                if (nums1[i - 1] == nums2[j - 1]) {
                    f[i][j] = f[i - 1][j - 1] + 1;
                } else {
                    f[i][j] = max(f[i - 1][j], f[i][j - 1]);
                }
            }
        }
        return f[m][n];
    }
};

Go

func maxUncrossedLines(nums1 []int, nums2 []int) int {
	m, n := len(nums1), len(nums2)
	f := make([][]int, m+1)
	for i := range f {
		f[i] = make([]int, n+1)
	}
	for i := 1; i <= m; i++ {
		for j := 1; j <= n; j++ {
			if nums1[i-1] == nums2[j-1] {
				f[i][j] = f[i-1][j-1] + 1
			} else {
				f[i][j] = max(f[i-1][j], f[i][j-1])
			}
		}
	}
	return f[m][n]
}

TypeScript

function maxUncrossedLines(nums1: number[], nums2: number[]): number {
    const m = nums1.length;
    const n = nums2.length;
    const f: number[][] = Array.from({ length: m + 1 }, () => Array(n + 1).fill(0));
    for (let i = 1; i <= m; ++i) {
        for (let j = 1; j <= n; ++j) {
            if (nums1[i - 1] === nums2[j - 1]) {
                f[i][j] = f[i - 1][j - 1] + 1;
            } else {
                f[i][j] = Math.max(f[i - 1][j], f[i][j - 1]);
            }
        }
    }
    return f[m][n];
}

JavaScript

/**
 * @param {number[]} nums1
 * @param {number[]} nums2
 * @return {number}
 */
var maxUncrossedLines = function (nums1, nums2) {
    const m = nums1.length;
    const n = nums2.length;
    const f = Array.from({ length: m + 1 }, () => Array(n + 1).fill(0));
    for (let i = 1; i <= m; ++i) {
        for (let j = 1; j <= n; ++j) {
            if (nums1[i - 1] === nums2[j - 1]) {
                f[i][j] = f[i - 1][j - 1] + 1;
            } else {
                f[i][j] = Math.max(f[i - 1][j], f[i][j - 1]);
            }
        }
    }
    return f[m][n];
};