Skip to content

Latest commit

 

History

History
376 lines (332 loc) · 9.68 KB

README_EN.md

File metadata and controls

376 lines (332 loc) · 9.68 KB
comments difficulty edit_url rating source tags
true
Hard
2170
Weekly Contest 249 Q3
Dynamic Programming

1931. Painting a Grid With Three Different Colors

中文文档

Description

You are given two integers m and n. Consider an m x n grid where each cell is initially white. You can paint each cell red, green, or blue. All cells must be painted.

Return the number of ways to color the grid with no two adjacent cells having the same color. Since the answer can be very large, return it modulo 109 + 7.

 

Example 1:

Input: m = 1, n = 1
Output: 3
Explanation: The three possible colorings are shown in the image above.

Example 2:

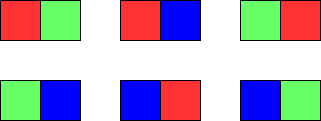
Input: m = 1, n = 2
Output: 6
Explanation: The six possible colorings are shown in the image above.

Example 3:

Input: m = 5, n = 5
Output: 580986

 

Constraints:

  • 1 <= m <= 5
  • 1 <= n <= 1000

Solutions

Solution 1: State Compression + Dynamic Programming

We notice that the number of rows in the grid does not exceed $5$, so there are at most $3^5=243$ different color schemes in a column.

Therefore, we define $f[i][j]$ to represent the number of schemes in the first $i$ columns, where the coloring state of the $i$th column is $j$. The state $f[i][j]$ is transferred from $f[i - 1][k]$, where $k$ is the coloring state of the $i - 1$th column, and $k$ and $j$ meet the requirement of different colors being adjacent. That is:

$$ f[i][j] = \sum_{k \in \textit{valid}(j)} f[i - 1][k] $$

where $\textit{valid}(j)$ represents all legal predecessor states of state $j$.

The final answer is the sum of $f[n][j]$, where $j$ is any legal state.

We notice that $f[i][j]$ is only related to $f[i - 1][k]$, so we can use a rolling array to optimize the space complexity.

The time complexity is $O((m + n) \times 3^{2m})$, and the space complexity is $O(3^m)$. Here, $m$ and $n$ are the number of rows and columns of the grid, respectively.

Python3

class Solution:
    def colorTheGrid(self, m: int, n: int) -> int:
        def f1(x: int) -> bool:
            last = -1
            for _ in range(m):
                if x % 3 == last:
                    return False
                last = x % 3
                x //= 3
            return True

        def f2(x: int, y: int) -> bool:
            for _ in range(m):
                if x % 3 == y % 3:
                    return False
                x, y = x // 3, y // 3
            return True

        mod = 10**9 + 7
        mx = 3**m
        valid = {i for i in range(mx) if f1(i)}
        d = defaultdict(list)
        for x in valid:
            for y in valid:
                if f2(x, y):
                    d[x].append(y)
        f = [int(i in valid) for i in range(mx)]
        for _ in range(n - 1):
            g = [0] * mx
            for i in valid:
                for j in d[i]:
                    g[i] = (g[i] + f[j]) % mod
            f = g
        return sum(f) % mod

Java

class Solution {
    private int m;

    public int colorTheGrid(int m, int n) {
        this.m = m;
        final int mod = (int) 1e9 + 7;
        int mx = (int) Math.pow(3, m);
        Set<Integer> valid = new HashSet<>();
        int[] f = new int[mx];
        for (int i = 0; i < mx; ++i) {
            if (f1(i)) {
                valid.add(i);
                f[i] = 1;
            }
        }
        Map<Integer, List<Integer>> d = new HashMap<>();
        for (int i : valid) {
            for (int j : valid) {
                if (f2(i, j)) {
                    d.computeIfAbsent(i, k -> new ArrayList<>()).add(j);
                }
            }
        }
        for (int k = 1; k < n; ++k) {
            int[] g = new int[mx];
            for (int i : valid) {
                for (int j : d.getOrDefault(i, List.of())) {
                    g[i] = (g[i] + f[j]) % mod;
                }
            }
            f = g;
        }
        int ans = 0;
        for (int x : f) {
            ans = (ans + x) % mod;
        }
        return ans;
    }

    private boolean f1(int x) {
        int last = -1;
        for (int i = 0; i < m; ++i) {
            if (x % 3 == last) {
                return false;
            }
            last = x % 3;
            x /= 3;
        }
        return true;
    }

    private boolean f2(int x, int y) {
        for (int i = 0; i < m; ++i) {
            if (x % 3 == y % 3) {
                return false;
            }
            x /= 3;
            y /= 3;
        }
        return true;
    }
}

C++

class Solution {
public:
    int colorTheGrid(int m, int n) {
        auto f1 = [&](int x) {
            int last = -1;
            for (int i = 0; i < m; ++i) {
                if (x % 3 == last) {
                    return false;
                }
                last = x % 3;
                x /= 3;
            }
            return true;
        };
        auto f2 = [&](int x, int y) {
            for (int i = 0; i < m; ++i) {
                if (x % 3 == y % 3) {
                    return false;
                }
                x /= 3;
                y /= 3;
            }
            return true;
        };

        const int mod = 1e9 + 7;
        int mx = pow(3, m);
        unordered_set<int> valid;
        vector<int> f(mx);
        for (int i = 0; i < mx; ++i) {
            if (f1(i)) {
                valid.insert(i);
                f[i] = 1;
            }
        }
        unordered_map<int, vector<int>> d;
        for (int i : valid) {
            for (int j : valid) {
                if (f2(i, j)) {
                    d[i].push_back(j);
                }
            }
        }
        for (int k = 1; k < n; ++k) {
            vector<int> g(mx);
            for (int i : valid) {
                for (int j : d[i]) {
                    g[i] = (g[i] + f[j]) % mod;
                }
            }
            f = move(g);
        }
        int ans = 0;
        for (int x : f) {
            ans = (ans + x) % mod;
        }
        return ans;
    }
};

Go

func colorTheGrid(m int, n int) (ans int) {
	f1 := func(x int) bool {
		last := -1
		for i := 0; i < m; i++ {
			if x%3 == last {
				return false
			}
			last = x % 3
			x /= 3
		}
		return true
	}
	f2 := func(x, y int) bool {
		for i := 0; i < m; i++ {
			if x%3 == y%3 {
				return false
			}
			x /= 3
			y /= 3
		}
		return true
	}
	mx := int(math.Pow(3, float64(m)))
	valid := map[int]bool{}
	f := make([]int, mx)
	for i := 0; i < mx; i++ {
		if f1(i) {
			valid[i] = true
			f[i] = 1
		}
	}
	d := map[int][]int{}
	for i := range valid {
		for j := range valid {
			if f2(i, j) {
				d[i] = append(d[i], j)
			}
		}
	}
	const mod int = 1e9 + 7
	for k := 1; k < n; k++ {
		g := make([]int, mx)
		for i := range valid {
			for _, j := range d[i] {
				g[i] = (g[i] + f[j]) % mod
			}
		}
		f = g
	}
	for _, x := range f {
		ans = (ans + x) % mod
	}
	return
}

TypeScript

function colorTheGrid(m: number, n: number): number {
    const f1 = (x: number): boolean => {
        let last = -1;
        for (let i = 0; i < m; ++i) {
            if (x % 3 === last) {
                return false;
            }
            last = x % 3;
            x = Math.floor(x / 3);
        }
        return true;
    };
    const f2 = (x: number, y: number): boolean => {
        for (let i = 0; i < m; ++i) {
            if (x % 3 === y % 3) {
                return false;
            }
            x = Math.floor(x / 3);
            y = Math.floor(y / 3);
        }
        return true;
    };
    const mx = 3 ** m;
    const valid = new Set<number>();
    const f: number[] = Array(mx).fill(0);
    for (let i = 0; i < mx; ++i) {
        if (f1(i)) {
            valid.add(i);
            f[i] = 1;
        }
    }
    const d: Map<number, number[]> = new Map();
    for (const i of valid) {
        for (const j of valid) {
            if (f2(i, j)) {
                d.set(i, (d.get(i) || []).concat(j));
            }
        }
    }
    const mod = 10 ** 9 + 7;
    for (let k = 1; k < n; ++k) {
        const g: number[] = Array(mx).fill(0);
        for (const i of valid) {
            for (const j of d.get(i) || []) {
                g[i] = (g[i] + f[j]) % mod;
            }
        }
        f.splice(0, f.length, ...g);
    }
    let ans = 0;
    for (const x of f) {
        ans = (ans + x) % mod;
    }
    return ans;
}